Directivity and Antenna Gain
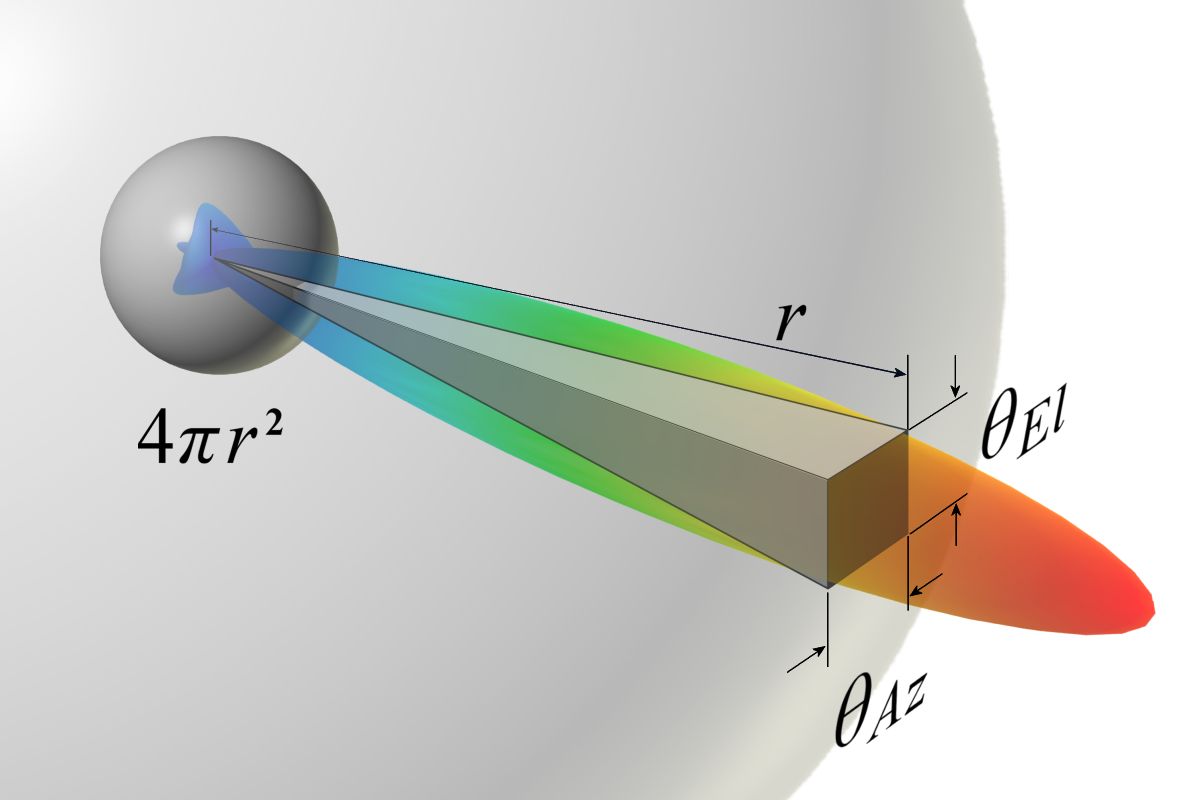
Figure 1: Comparison of the surface illuminated by the directional antenna with a spherical surface,
(Note: the radius of the sphere must be equal to the distance of the rectangle from the center point when comparing).

Figure 1: Comparison of the surface illuminated by the directional antenna with a spherical surface,
(Note: the radius of the sphere must be equal to the distance of the rectangle from the center point when comparing).
Directivity and Antenna Gain
Antenna gain G describes the directivity D and efficiency η of an antenna as an easy to handle parameter.
Directivity of an Antenna
The directivity of an antenna is the ratio of the power density S (radiant intensity per unit area) of the real antenna in its main direction to a hypothetical but non-existent isotropic radiator that would radiate uniformly in all directions. The power density of the isotropic radiator is thus the radiated power evenly distributed on a sphere surface. Every real antenna has a more or less strong directivity. The directivity is defined by the ratio of the power density of the real antenna in its main direction to the power density of the isotropic omni-directional antenna:
| D = | S |
S = power density of a real antenna Si = power density of the isotropic radiator |
(1) | |
| Si |
A directional antenna concentrates its radiated power only on a small part of the sphere surface. This area fraction can also be set in relation to the entire surface of the sphere. This area fraction is calculated using the radians of the two half-power beam-widths. (In this radian measure is also the radius of the unit circle. These shorten with the radius of the sphere surface.) The height and width of this effective area are defined as multiples of the wavelength used.
| D = | Asphere | ≅ | 4π r2 | = | 4π | = | 4π · Aeff |
Asphere = entire surface of a sphere Aeff = b·h = effective aperture on the surface of the sphere θAz = half-power beamwidth in azimuth (in radians) θEl = half-power beamwidth in elevation (in radians) b = surface width h = surface height |
(2) | |
| Aeff | θAz · θEl | λ/b · λ/h | λ2 |
Here, however, inaccuracy is included, because surface Aeff = b·h is at one plane and not at sphere-surface. This inaccuracy is all the smaller, the smaller the area b·h compared to the sphere surface is, the better the directional characteristic of the antenna is. This inaccuracy can be ignored at half-power beam-widths of up to 20°.
The Gain of an Antenna
The directivity is an essential component of antenna gain. In the case of a real antenna gain, gains and losses must be considered. The radiated power of an antenna is proportional for each antenna to the fed-in transmitter power, which can easily be measured on the feed line to the antenna. However, part of transmitters power is lost within the antenna on the feed line at its ohmic resistances. These losses are defined as the efficiency of an antenna. If there were an ideal antenna without these losses, the antenna gain would be equal to its directivity.
The antenna gain is smaller by the efficiency factor than the directivity:
| G = η· D | (3) |
An antenna gain does not always have to be greater than 1. There are also antennas with an antenna gain of less than 1. These are usually measurement antennas where it is important to achieve a uniform antenna gain over a very large frequency range in order to compare the measurement results from different frequency bands.
Simplifications
Some simplifications have to be made in order to be able to relate the spherical surface of an isotropic radiator to the effective aperture of an antenna:
- It is assumed that all radiated energy is concentrated in the main lobe of the antenna. So there would be no sidelobes.
- It is assumed that all radiated energy of the antenna is concentrated within the measured and propagated half-power beamwidth of the antenna. This means that there is no radiated energy outside this beamwidth.
- The energy is evenly distributed within the half-power beamwidth. (Thus a rather cone-shaped figure becomes a pyramid-shaped one. The rectangle occurs with the dimensions half-power beamwidth in elevation multiplied with half-power beamwidth in azimuth!)
The two geometric surfaces can only be compared with each other because of these assumed simplifications.
